S.I. No. 416/2004 -- Asset Covered Securities Act, 2001 (Section 91(1)) (Sensitivity To Interest Rate Changes) Regulation, 2004
|
ASSET COVERED SECURITIES ACT, 2001 (SECTION 91(1)) (SENSITIVITY TO INTEREST RATE CHANGES) REGULATION, 2004 |
||||||
|
ASSET COVERED SECURITIES ACT, 2001 (SECTION 91(1)) (SENSITIVITY TO INTEREST RATE CHANGES) REGULATION, 2004 |
||||||
|
Section 33C of the Central Bank Act, 1942 (as amended) designates the Irish Financial Services Regulatory Authority (the “Authority”) as being the body responsible for exercising the powers conferred on the Central Bank and Financial Services Authority of Ireland by Section 91 (1) of the Asset Covered Securities Act, 2001 (No. 47 of 2001). The Authority hereby makes the following Regulation: 1. This Regulation may be called the Asset Covered Securities Act, 2001 (Section 91 (1)) (Sensitivity to Interest Rate Changes) Regulation, 2004. 2. In this Regulation the “Act” means the Asset Covered Securities Act, 2001 (No. 47 of 2001). A word or expression that is used in this Regulation and is also used in the Act has, unless the contrary intention appears, the same meaning in this Regulation as in the Act. 3. Measurement of sensitivity to interest rate changes shall be determined as follows |
||||||
|
(a) Sensitivity to interest rate changes shall be calculated using net present value changes based on:- |
||||||
|
One hundred basis points upward shift in the yield curve |
||||||
|
One hundred basis points downward shift in the yield curve and |
||||||
|
One hundred basis points twist in the yield curve. |
||||||
|
(b) All calculations of sensitivity to interest rate changes are to be carried out in accordance with the formulae set out in the Schedule to this Regulation. |
||||||
|
(c) All positions sensitive to changes in interest rates (whether in the cover-assets pool or not), but excluding capital, should be included in the measurement. 4. Net present value changes arising from any of the scenarios above shall not exceed 10% of the designated mortgage credit institution's total own funds at any time. |
||||||
|
||||||
|
EXPLANATORY NOTE |
||||||
|
This note is not part of the Instrument and does not purport to be a legal interpretation. |
||||||
|
This Regulation prescribes formulae for calculation of sensitivity to interest rate changes for designated mortgage credit institutions. |
||||||
|
SCHEDULE |
||||||
|
FORMULAE IN RESPECT OF SECTION 91(1) (SENSITIVITY TO INTEREST RATE CHANGES) REGULATION |
||||||
|
This schedule sets out the formulae to be used by designated mortgage credit institutions in measuring sensitivity to interest rate changes. |
||||||
|
1. Calculation of Net Present Values |
||||||
|
1.1 Fixed Interest Rate Cover Assets |
||||||
|
The net present value of a cover asset, the interest payable on which is fixed, is calculated as the sum of the present values of each cashflow payable on the instrument in each time period: |
||||||
|
|
||||||
|
where |
||||||
|
CFj cash flow |
||||||
|
f the number of cash flows per year |
||||||
|
j/f the time in years to the jth cash flow |
||||||
|
N the number of cash flows during the fixed-rate period |
||||||
|
sj the annualised Euribor or Libor zero-coupon rate to maturity j/f |
||||||
|
z a constant spread to the Euribor or Libor zero coupon rate that discounts the cash flows of an at-market loan, deposit or security of maturity N / f to par |
||||||
|
PN the principal outstanding just after receipt of the Nth cash flow. (If the loan is fixed to maturity, PN =0) |
||||||
|
1.2 Floating Interest Rate Cover Assets |
||||||
|
The net present value of a cover asset, the interest payable on which is floating, is calculated as the sum of the present values of each cashflow payable on the instrument in each time period: |
||||||
|
|
||||||
|
where |
||||||
|
Po is principal outstanding at the valuation date, time zero |
||||||
|
t time in years (or part of a year) to the next re-pricing date |
||||||
|
r is the annualised rate on the asset or liability on a simple interest basis |
||||||
|
St the annualised Euribor or Libor zero-coupon rate to maturity t |
||||||
|
x a constant spread to the Euribor or Libor zero coupon rate that discounts the cash flows of an equivalent at-market loan, deposit or security to par |
||||||
|
1.3 Fixed Interest Rate Cover Asset Hedge Contracts or Securities Issued |
||||||
|
The net present value of a cover asset hedge contract or security issued, the interest payable on which is fixed, is calculated as the sum of the present values of each cashflow payable on the instrument in each time period: |
||||||
|
|
||||||
|
where |
||||||
|
CFt = Interestt + Capitalt |
||||||
|
st is the zero coupon interest rate, at valuation, to time t |
||||||
|
z is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
1.4 Floating Interest Rate Cover Asset Hedge Contracts or Securities Issued |
||||||
|
The net present value of a cover asset hedge contract or security issued, the interest payable on which is floating, is calculated as the sum of the present values of each cashflow payable on the instrument in each time period: |
||||||
|
|
||||||
|
where |
||||||
|
CFt = Interestt + Capitalt; |
||||||
|
st is the zero coupon interest rate, at valuation, to time t; and |
||||||
|
x is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
1.5 Net Present Value of Cover Asset Pool or Securities Issued |
||||||
|
For the purposes of this regulation, the net present value of the cover asset pool or securities issued is calculated as the sum of the net present values of the instruments in the cover assets pool or the securities issued as the case may be. |
||||||
|
1.6 Cover Assets Hedge Contracts |
||||||
|
Where a cover assets hedge contract has a notional amount equal to the outstanding principal or nominal from time to time of mortgage credit assets and/or substitution assets which are cover assets from time to time and that cover assets hedge contract has the hedging effect of converting a blended or averaged interest rate, (determined by reference to various interest rates payable on those cover assets) into a single floating or fixed rate, that cover assets hedge contract shall be treated for the net present value purposes and for the purpose of this regulatory notice together with the related mortgage credit assets and/or substitution assets as a single floating rate asset or, as applicable, single fixed rate asset. |
||||||
|
Where a cover assets hedge contract has a notional amount equal to the outstanding principal (or nominal) and term of one or more issues of mortgage covered securities and that cover assets hedge contract has the hedging effect of converting the interest rate payable on those mortgage covered securities into a single floating or fixed rate, that cover assets hedge contract shall be treated for the net present value purposes and for the purpose of this regulatory notice together with the related mortgage covered securities as a single floating rate asset or, as applicable, single fixed rate asset. |
||||||
|
The net present value of any other cover asset hedge contract should be calculated, as appropriate, on the basis of the applicable formulae set out above in section 1.3 or 1.4 above. |
||||||
|
2. Measurement of Sensitivity to Interest Rate Changes |
||||||
|
Designated mortgage credit institutions shall use the following methodology in measuring their sensitivity to changes in interest rates. |
||||||
|
2.1 Upward Parallel Shift in the Yield Curve |
||||||
|
For a one hundred basis points upward shift in the yield curve, the resultant net present value of the cover asset pool, securities issued or other positions sensitive to changes in interest rates shall be calculated as: |
||||||
|
|
||||||
|
where, for an individual cover asset or cover asset hedge contract, i: |
||||||
|
|
||||||
|
ySU,t = Max[(st + z) + (0.01),0] for fixed rate instruments; |
||||||
|
ySU,t = Max[(st + x) + (0.01),0] for floating rate instruments; |
||||||
|
st = zero coupon interest rate, at valuation to time t; |
||||||
|
z is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price; and |
||||||
|
x is the static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
2.2 Downward Parallel Shift in the Yield Curve |
||||||
|
For a one hundred basis points downward shift in the yield curve, the resultant net present value of the cover asset pool, securities issued or other positions sensitive to changes in interest rates shall be calculated as: |
||||||
|
|
||||||
|
where, for an individual cover asset or cover asset hedge contract, i: |
||||||
|
|
||||||
|
ySD,t = Max[(st + z) - (0.01),0] for fixed rate instruments; |
||||||
|
ySD,t = Max[(st + x) - (0.01),0] for floating rate instruments; |
||||||
|
st = zero coupon interest rate, at valuation, to time t, |
||||||
|
z is the static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price; and |
||||||
|
x is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
2.3 Changes in the Slope of the Yield Curve |
||||||
|
2.3.1 General Approach |
||||||
|
The procedure for the calculation of the net present value of the cover asset pool, securities issued or other positions sensitive to changes in interest rates resulting from a one hundred basis points twist in the yield curve is as follows: |
||||||
|
• For all revaluation points along the yield curve up to and including 3 months (i.e. the ‘short end’), increase (decrease) the yield curve values by 100 basis points; |
||||||
|
• For all revaluation points along the yield curve greater than or equal to 10 years (i.e. the ‘long end’), decrease (increase) the yield curve values by 100 basis points; and |
||||||
|
• In the interval from 3 months to 10 years, make proportional changes to the yield curve in accordance with the number of revaluation points in the interval. |
||||||
|
This new yield curve is then applied to all instruments in the cover asset pool, securities issued or other positions sensitive to changes in interest rates. The formulae for the calculation of the net present value of all instruments resulting from a one hundred basis points twist in the yield curve are set out in 2.3.2 and 2.3.3 below. |
||||||
|
2.3.2 Downward Change in the Slope of the Yield Curve |
||||||
|
For a twist in the yield curve, up one hundred basis points in the short term and down one hundred basis points in the long term, with proportional changes in the interval, the resultant net present value of the cover asset pool, securities issued or other positions sensitive to changes in interest rates shall be calculated as: |
||||||
|
|
||||||
|
where, for an individual cover asset or cover asset hedge contract, i: |
||||||
|
|
||||||
|
|
||||||
|
|
||||||
|
α, β ≥ 1; α, β € N; |
||||||
|
α = 1 for t ≤ 3 months; |
||||||
|
α = β for t ≥ 10 years; |
||||||
|
for 3 months ≤ t ≤ 10 years, assign ascending values of α to each revaluation point along the curve greater than 3 months and less than 10 years, beginning with α = 1 for t = 3 months; |
||||||
|
β is the final revaluation point along the curve required in the interval (3 months ≤ t ≤ 10 years); |
||||||
|
z is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price; and |
||||||
|
x is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
2.3.3 Upward Change in the Slope of the Yield Curve |
||||||
|
For a twist in the yield curve, down one hundred basis points in the short term and up one hundred basis points in the long term, with proportional changes in the interval, the resultant net present value of cover asset pool, securities issued or other positions sensitive to changes in interest rates shall be calculated as: |
||||||
|
|
||||||
|
where, for an individual cover asset or cover asset hedge contract, i: |
||||||
|
|
||||||
|
|
||||||
|
|
||||||
|
α, β ≥ 1; α, β € N; |
||||||
|
α = 1 for t ≤ 3 months; |
||||||
|
α = β for t ≥ 10 years; |
||||||
|
for 3 months ≤ t ≤ 10 years, assign ascending values of α to each revaluation point along the curve greater than 3 months and less than 10 years, beginning with α = 1 for t = 3 months; |
||||||
|
β is the final revaluation point along the curve required in the interval (3 months ≤ t ≤ 10 years); |
||||||
|
z is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price; and |
||||||
|
x is a static spread over the zero coupon interest rate that lets the net present value of the instrument be equal to its price. |
||||||
|
2.4 Sensitivity to changes in interest rates |
||||||
|
For the purposes of this Regulation, sensitivity to changes in interest rates is measured as the difference between: |
||||||
|
• Actual net present value and the resultant net present value from a one hundred basis point upward shift in the yield curve; |
||||||
|
• Actual net present value and the resultant net present value from a one hundred basis point downward shift in the yield curve; |
||||||
|
• Actual net present value and the resultant net present value from a twist in the yield curve of plus one hundred basis points in the short term and minus one hundred basis points in the long term, with proportional changes in the interval; and |
||||||
|
• Actual net present value and the resultant net present value from a twist in the yield curve of minus one hundred basis points in the short term and plus one hundred basis points in the long term, with proportional changes in the interval. |
||||||
|
3. Reference Interest-Rate Curve |
||||||
|
For the purposes of this schedule, the appropriate treasury curve for each currency should be used as the reference curve for valuing all cover assets, cover asset hedge contracts and securities issued. For the purposes of the Euro currency, the Euro-Swap Curve should be used. |
||||||


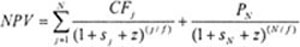
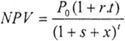
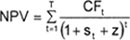
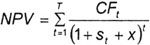
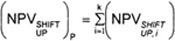
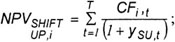
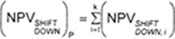
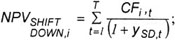
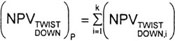
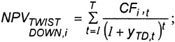
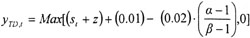 for fixed rate instruments;
for fixed rate instruments;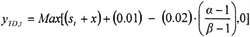 for floating rate instruments;
for floating rate instruments;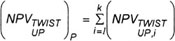
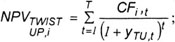
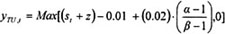 for fixed rate instruments;
for fixed rate instruments;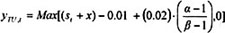 for floating rate instruments;
for floating rate instruments;